What Are Intervals?

What are intervals?

“Interval” is simply the term we use in music when we want to measure the distance between two notes.  There are two components to identifying intervals. The first is the quality, whether the interval is major, minor, perfect, augmented, or diminished. The second is the number, whether the interval is a distance of a second, third, fourth, etc.

We’ll start by identifying all of the diatonic intervals of the major scale, which refers to the individual intervals within a major scale, starting at the root and measuring up to each degree of the scale.

As you can see, in the diatonic major scale we are dealing with two different qualities: major and perfect.

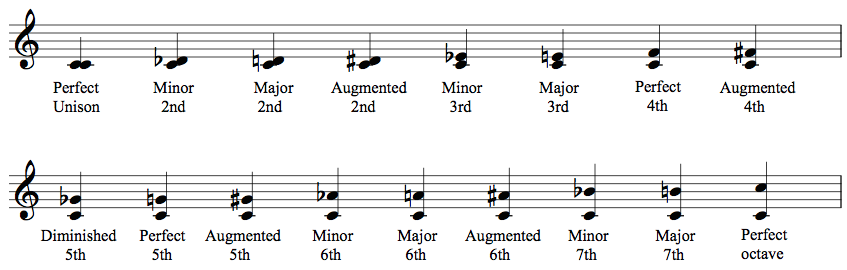
Of course, the diatonic major scale does not include the chromatic intervals. In order to see every interval within the span of an octave we need to start at a given root (in our example, we’ll use ‘C’) and measure up the octave by increasing a half-step at a time. Doing so results in the following:

The above graphic shows the distance between middle ‘C’ and every note within the octave, each time adding a half-step and identifying the resulting interval. There is something very important to notice about this graphic however – some of the intervals are repeats of one another, but they are spelled differently. Look closely at the “augmented 2nd” interval and the “minor 3rd” interval. What do you notice?

You should have noticed that ‘D#’ and ‘Eb’ are technically the same note – the only difference is that they are spelled differently. In music we call this alternate spelling of the same tone an enharmonic spelling. But if the interval between C and D# is the same interval as that between C and Eb, why do we call one an augmented 2nd and the other a minor 3rd? Why two different names if they are both indicating the same interval?

The answer goes back to the beginning of this article. An interval has 2 components – the quality, and the number (or distance). The distance between C and D is some sort of 2nd. It could be a minor 2nd, major 2nd, or augmented 2nd (referring to the quality), but the distance between any ‘C’ (i.e., C-natural, C-sharp, or C-flat) and any ‘D’ (D-natural, D-sharp, D-flat) is always a 2nd. Once we determine the number/distance, we then identify the quality (major, minor, etc). The distance between C and D# is an augmented 2nd. The distance between C and E is some sort of third, and in this instance the E-flat means that the 3rd is a minor 3rd.

Look at some other intervals, such as the augmented 4th and diminished 5th, the augmented 5th and minor 6th, and the augmented 6th and minor 7th, for more examples of enharmonic spellings.

Becoming more familiar with intervals and being able to quickly identify intervals is very helpful to the skill of arranging/composing, improvisation, transposition, and harmonic analysis. You can practice interval identification by simply sitting at the piano, playing two notes (within an octave), and identifying the interval. Be sure to practice in all 12 keys.